Introduction
What can you do with a PocketLab Mini HotRod, Voyager, five pieces of HotWheels track, and a half-dozen wood blocks about the size of Jenga blocks? How about an experiment in energy conservation! Add CloudLab and you have an environment for your students/lab groups to perform, analyze, document and save their PocketLab lab reports.
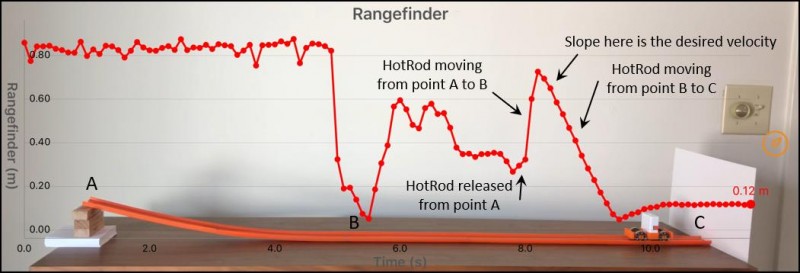
Figure 1 shows the lab setup for this lesson. The track consists of 5 pieces of HotWheels track, with Voyager and the Mini HotRod released from rest at point A. Two pieces of track from points A to B are free to move upward as the ramp's height (the independent variable) is allowed to vary from 1 to 5 Jenga blocks. Three pieces of track from points B to C are fastened to the horizontal tabletop with Velcro strips, keeping them stationary. The position of Voyager and the Mini HotRod while traveling to the right is captured by the rangefinder. A piece of white cardboard at the end of the track (point C) serves as the reference point for the Voyager's IR sensor.
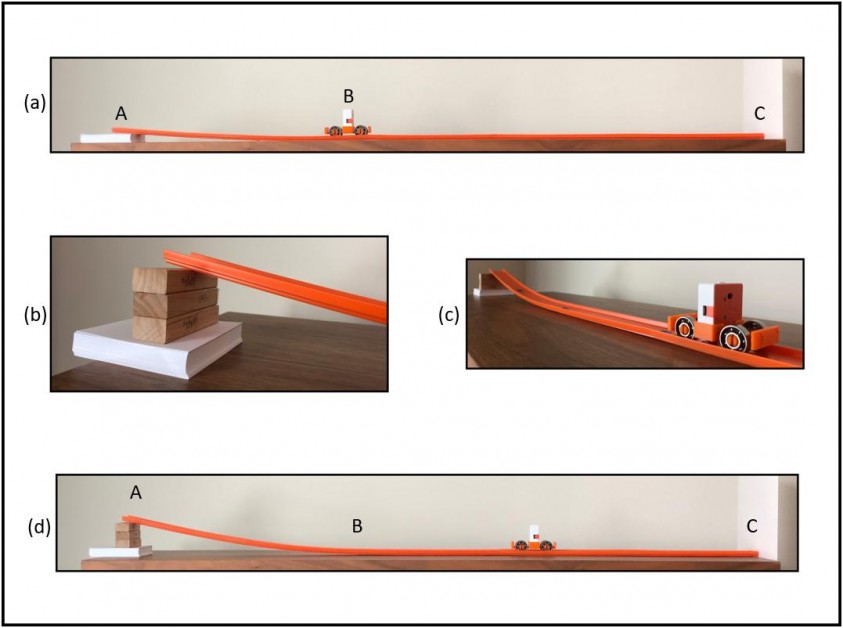
You will notice that a stack of small paper sheets is below the Jenga blocks in all of the images in Figure 1. The importance of this in the success of this experiment cannot be overemphasized. The height of this stack of paper sheets (when no Jenga blocks are present) is adjusted so that when the Mini HotRod is released, the HotRod will come to rest very close to point B. This height represents the amount of gravitational potential that is lost due to frictional forces such as bearing friction and friction between the wheels and track. This may be thought of as the "zero" of height of the ramp. We make the reasonable assumption that this is the amount of energy lost to friction, regardless of the height of the ramp. We keep this stack of paper sheets present throughout the experiment.
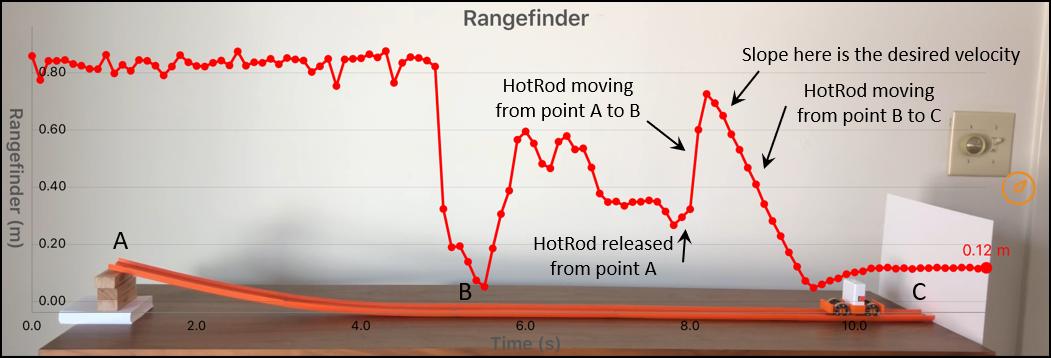
We are interested in determining the velocity (the dependent variable) of the cart at point B, when released from rest at point A, while varying the number of Jenga blocks from 1 to 5. In each case, the velocity can be determined from the the slope of the rangefinder vs. time graph at point B. Figure 2 is an aid to understanding a typical graph. It should be noted that the only meaningful rangefinder positions are those when Voyager is perpendicular to the white card on the far right, i.e., from point B to point C. At all other locations the rangefinder is not pointing directly at the white card.
Energy Conservation
Since we have adjusted for energy losses due to friction, the gravitational potential energy at the top of the ramp should equal the kinetic energy of the HotRod at point B, based on energy conservation:
where m is the mass of the HotRod plus Voyager, h is the height of the ramp, and v is the velocity of the HotRod at point B. Since m cancels out in the equation, a little bit of algebra quickly reveals that:
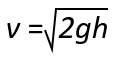
In other words, the velocity of the HotRod is proportional to the square root of the height. If we can obtain such a proportionality in our experimental results, then we have significant support for energy conservation.
CloudLab
If you are not familiar with CloudLab, it is strongly suggested that you read the short article "The CloudLab Model" before proceeding. It will give you an overview of the structure of CloudLab.
We now take you on a tour of CloudLab via a series of screenshots taken after our experiment was completed. Each screen shot is accompanied by a short discussion explaining the screenshot.
The top of Figure 3 shows the title of our Lab Report. Our experiment consisted of 5 runs. Each of the runs was given a name representing the height of the ramp in blocks. Each of the runs consisted of three trials. You can see that it is quite easy to add additional runs by clicking the green "Add Another Run" button. If we click on the down-arrow to the far right of the "Height = 1 Block" run, we can then see the details of that run.
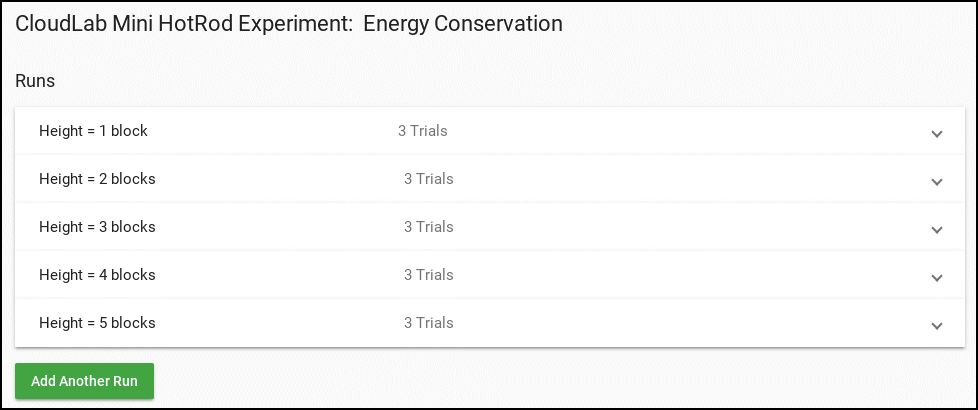
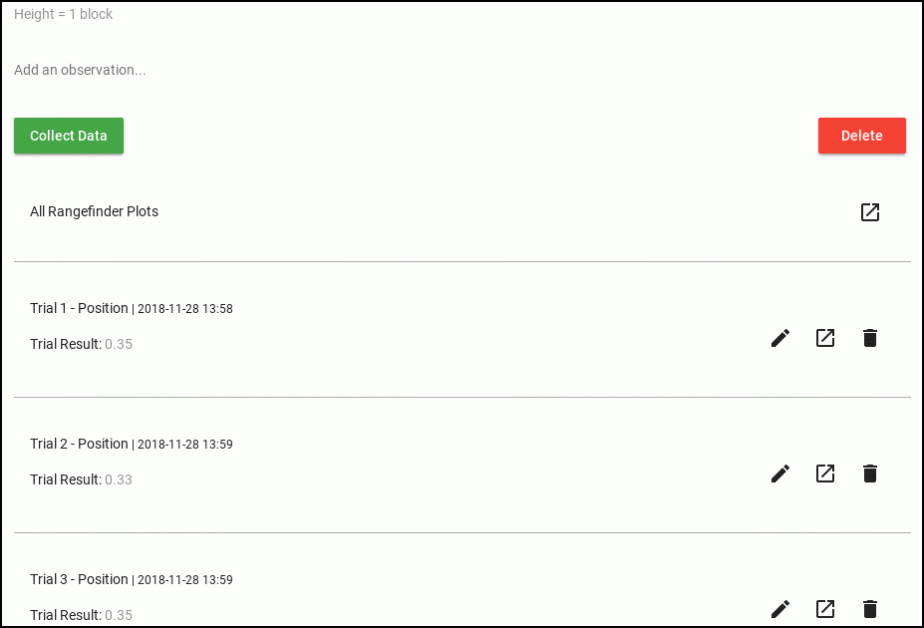
Figure 4 shows a summary of the three trials for the "Height = 1 Block" run. The "Trial Results" are the velocities of the the Mini HotRod at point B in Figure 1. You can see that the velocities are nearly the same for each of the three trials. The implication of this for the teacher is that the number of trials for each run in this experiment could have been one instead of three. This is a great way to reduce the amount of time required for students to do this experiment. At the far right of each trial are three icons. The pencil icon allows the student to enter the trial result. The middle icon opens up the graph of the data recorded by Voyager for that run. The trash can icon lets you discard a faulty trial.
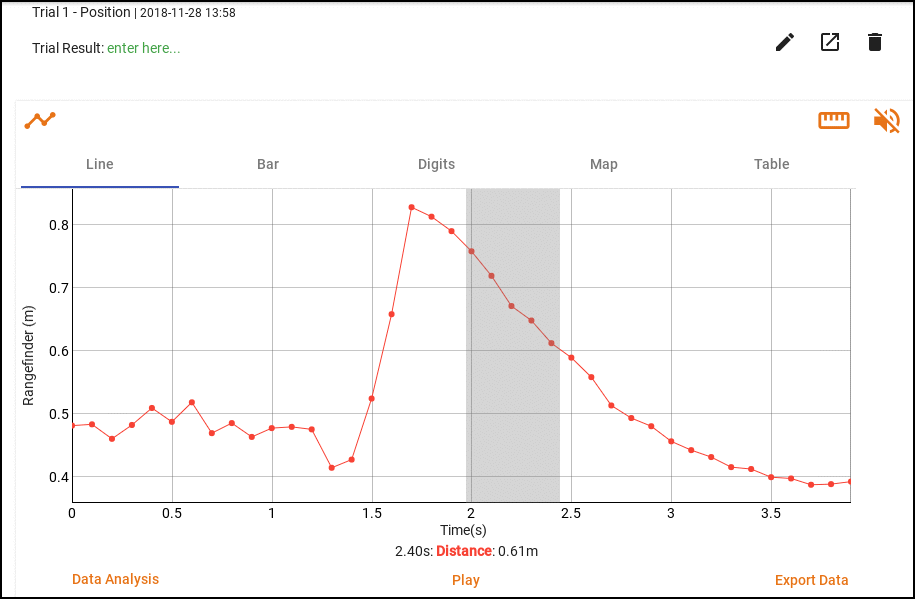
Let's now look at the graph of the data recorded in Trial 1 of the "Height = 1 Block" run, as shown in Figure 5. At approximately 1.7 seconds the HotRod reaches point B and gradually slows down until it stops shortly after 3.5 seconds. We want the velocity close to point B before any noticeable slowing down. We drag and click to highlight five points between 2 and 2.5 seconds. The slope of that region should give us the desired velocity.
After dragging the highlighted region, we obtain a zoomed in graph of just that highlighted region, as shown in Figure 6. We click on the "Data Analysis" button and select the linear option for curve fitting. We see that the slope of the best fit equation is -0.35 m/s. Since we are interested only in the magnitude, we key in 0.35 as the "Trial Result".
This process is repeated for each of the three trials in each of the five runs. After this we are ready to set up the Results Table.
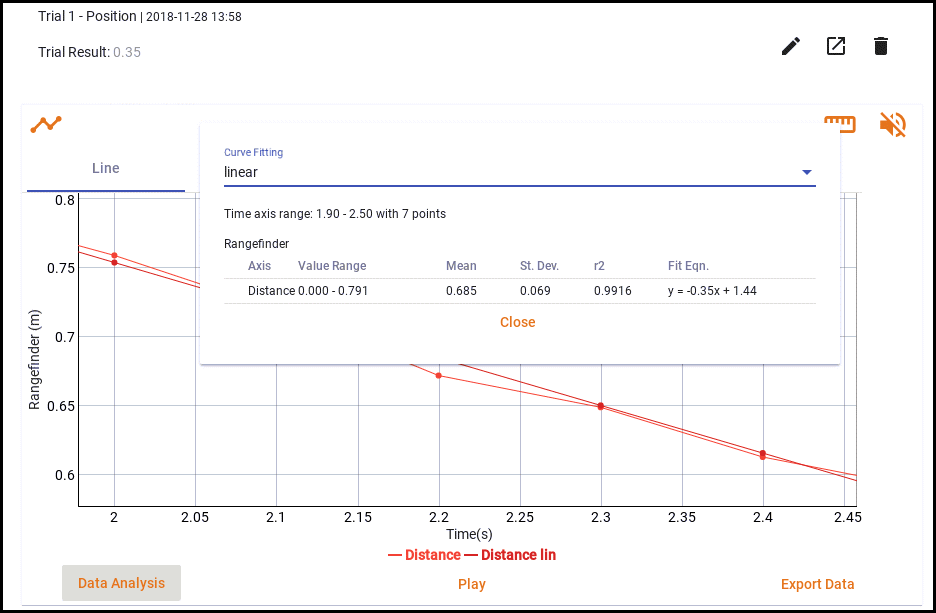
Figure 7 shows the results table in the Data Analysis Toolkit. The independent variable is keyed in as Height (blocks). The values 1 through 5 are then keyed in for the heights for each of the runs. The dependent variable is keyed in as Velocity (m/s). In this experiment, rather than using Manual Entry for the dependent variable, we click the radio button for "Trial Mean". For each of the five runs, CloudLab then calculates the mean value for the three trial results in each run and fills in those values automatically for us.
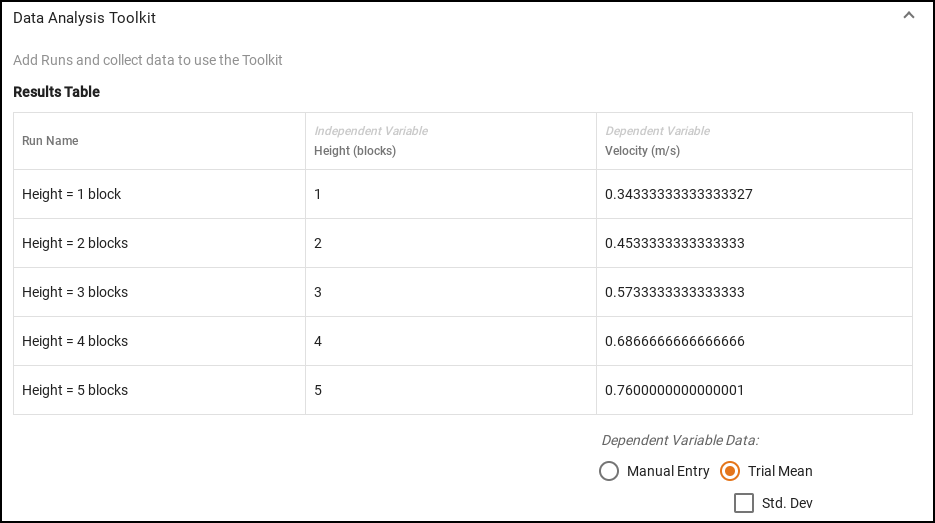
We can then work with the Results Graph, shown in Figure 8. A Bar graph will display by default, but we click on "Line" since we want a line graph. It is seen that the axes are automatically numbered and labeled in accordance with the Results Table of Figure 7.
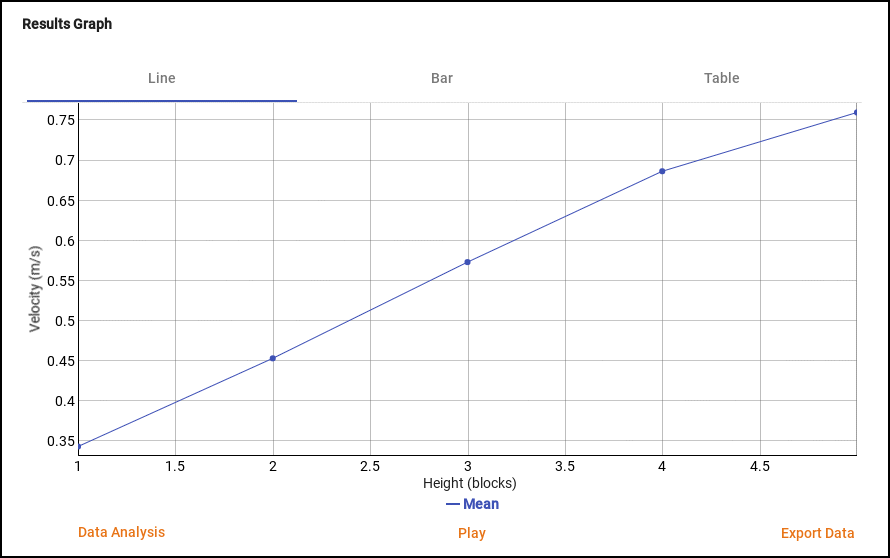
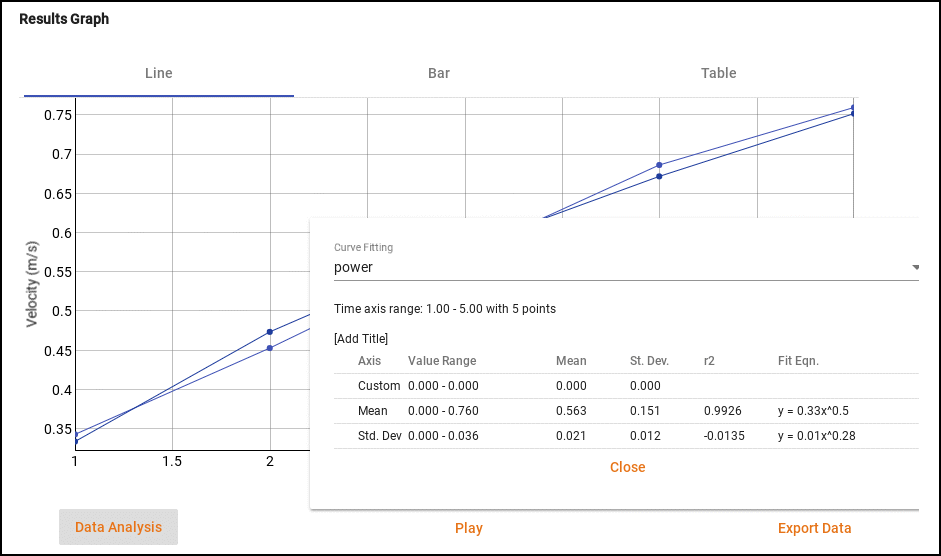
We are now ready for the data analysis of the results graph of Figure 8, so we click on "Data Analysis" in the lower left corner of the figure. Upon clicking the "Data Analysis" button and selecting power curve fitting, we obtain what is shown in Figure 9. We selected a power fit curve since energy conservation told us that velocity should be proportional to the square root of the height. Low and behold, the best fit power, as shown in the Fit Equation, is 0.5, i.e, square root!
Finally, we add a "Section" to our Lab Report for which we give the title "Conclusion", as shown in Figure 10. In the conclusion, we point out how our experiment gives strong support for energy conservation. Although we haven't done so, we could also include a You Tube video and an image. The ability to add hyperlinks is also planned. Additional "Sections" can be added by clicking on the "Add Another Section" button.