PocketLab makes is quite easy to investigate and verify the inverse cube law for the magnetic field of a neodymium magnet as a function of distance from the magnet. All that is needed in addition to The PocketLab is a centimeter ruler, small neodymium magnet, a small block of wood and a little double stick tape. The photo below shows how the neodymium magnet is taped to the block of wood with the magnet located at the 10 cm mark on the NSTA ruler. The height of the center of the magnet is at about the height of the circuit board inside of PocketLab. The X on the front face of PocketLab is very close to the location of the magnetic field sensor inside of PocketLab, 0.5 cm from the left edge of Pocket Lab, in line with the Y-axis of PocketLab.

The photo below shows the set up from above with the left edge of Pocket lab at the 15 cm mark. The distance between the dipole and the sensor is therefore about 5.5 cm in this photo.

In preparation for data collection, PocketLab is set to display magnetic field magnitude. It is then moved far from the neodymium magnet and zeroed. It is then placed at the 12 cm mark on the ruler, making the distance between magnet and sensor 2.5 cm. After a few seconds, PocketLab is moved to the 13 cm mark, thus increasing the distance by 1 cm to 3.5 cm. This process is continued through a distance of 8.5 cm. The magnetic field magnitude can be read directly from the movie, shown below, at each of the known distances.
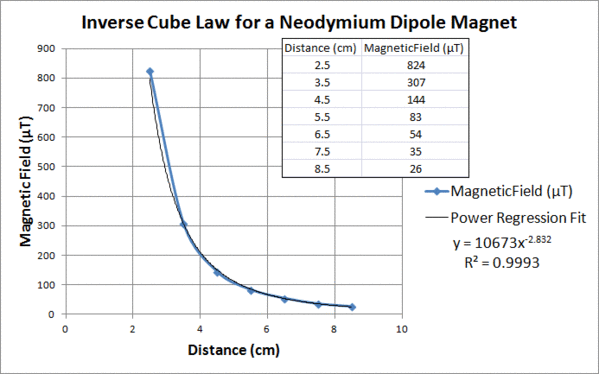
(Distance, magnetic field magnitude) data pairs are then entered into an Excel spreadsheet, and a chart of Magnetic Field vs. Distance is created. The chart, shown below, appears to show some sort of an inverse relationship between magnetic field and distance. The Excel "Add trendline" feature is then used and the "Power" regression fit is applied. It is found that the power is -2.832, very close to the -3 expected for an inverse cube relationship.
A copy of the Excel spreadsheet is included in the attachments for anyone interested in viewing it.

